09
-
9.1 Internal energy (U)
- In general energy can be categorised into groups, namely macroscopic and microscopic. The macroscopic forms of energy are those possessed by the system and depend on some outside reference frame. Examples are kinetic energy and potential energy. Microscopic forms of energy are related to the molecular structure of a system. The activity of the molecules manifests itself in various forms of microscopic energy such as molecular translation, rotation, vibration and electron spin and nuclear spin. The sum of all microscopic forms of energy in a system is called the internal energy U.
In general an increase in temperature lead to a higher internal energy due to the increase in molecular activity.
- Internal Energy (U) 📹
- The specific internal energy is defined as the amount of internal energy per unit mass of substance and is given the symbol u ( u = U | m). The SI unit for internal energy (U) and specific internal energy (u) are J or kJ and J/kg or kJ/kg respectively.
- In general energy can be categorised into groups, namely macroscopic and microscopic. The macroscopic forms of energy are those possessed by the system and depend on some outside reference frame. Examples are kinetic energy and potential energy. Microscopic forms of energy are related to the molecular structure of a system. The activity of the molecules manifests itself in various forms of microscopic energy such as molecular translation, rotation, vibration and electron spin and nuclear spin. The sum of all microscopic forms of energy in a system is called the internal energy U.
-
9.2 Enthalpy (H)
- The enthalpy of a homogeneous system is defined as the property of substance which combines the internal energy (U), Pressure (P) and Volume (V). The relationship can be expressed as:
H = U + PV
The enthalpy is an extensive property and it is convenient to introduce the specific enthalpy h ( h = H / m and h = u + Pv, where u is the specific internal energy, P is the pressure, and v is the specific volume). The SI unit for enthalpy (H) joule (J) and for specific enthalpy is joule per kilogram (J/kg).
- The enthalpy of a homogeneous system is defined as the property of substance which combines the internal energy (U), Pressure (P) and Volume (V). The relationship can be expressed as:
-
9.3 Work (W)
- As stated in section 7.2 the system can interact with its surroundings in three ways; work, heat and mass. Work (W) is a process by which a force (F) is moved through a distance (S).
W = F.S (Units: N.m=J)
The rate of work (power) is W = Work done / unit time [ Units: J | s = W (Watt) ]
Work is a transient form of energy or energy in transit. This means that it loses its identity as soon as enters or leaves a system and become part of the stored energy of a system.
- As stated in section 7.2 the system can interact with its surroundings in three ways; work, heat and mass. Work (W) is a process by which a force (F) is moved through a distance (S).
-
9.4 Heat (Q)
- Heat is an energy transfer process as a result of temperature difference between one body and another or a body and its surrounding.
Heat is like work it is a transient form of energy.
The rate of heat flow = Heat transfer / unit time
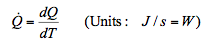
- Heat is an energy transfer process as a result of temperature difference between one body and another or a body and its surrounding.
-
9.5 Specific Heat Capacity(C)
- Specific heat capacity of a substance is defined as the amount of energy required to change the temperature of 1 kg of the substance by 1°C.
Therefore a substance specific heat capacity can be defined as the ratio of the amount of heat energy transferred to a substance and the resulting increase in temperature of the substance, Hence
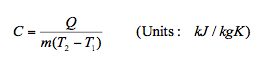
Different substances have different specific heat capacities. For example C water = 4.181kJ/kg, C Iron = 0.449kJ/kg and C Hydrogen = 14.304kJ/kg. Also specific heat capacity varies with temperature i.e. C = ⨍(T). Therefore in solving problems in thermodynamics it is usually given as an average value within a temperature range.
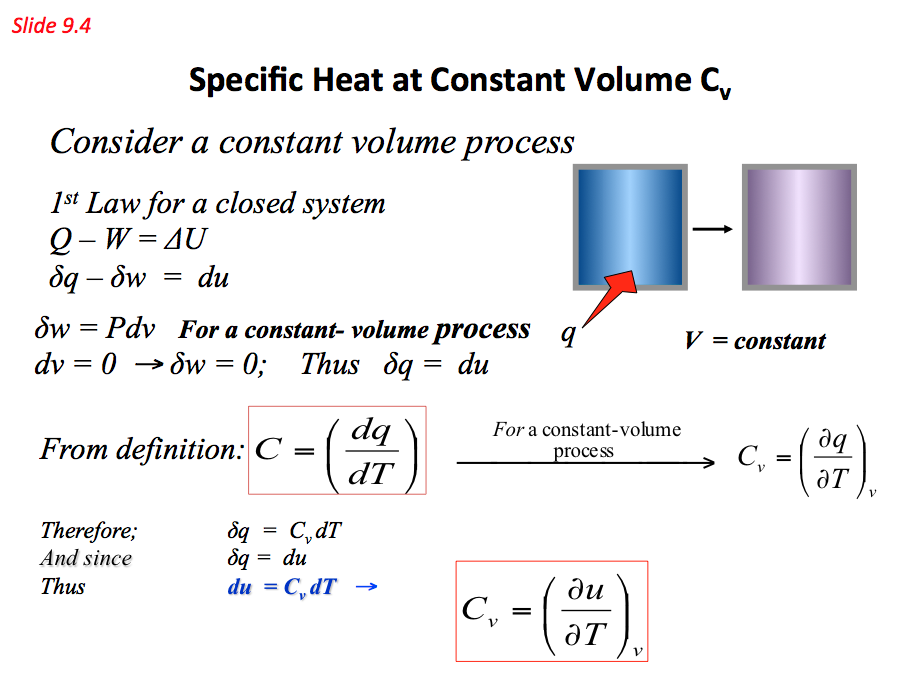
Beside the temperature, the heat capacity of a substance can be affected by many other state variables that describe the thermodynamics system under consideration such as the pressure and volume of the system before and after the addition of heat. As a result there are several slightly different measurements of heat capacity. The most common methods used are constant pressure (Cp) and constant volume (Cv). They can be found using
- Energy Transfer by Heat 📷
- Specific Heat 📷
- Specific Heat at Constant Volume Cv 📷
- Specific Heat at Constant Pressure Cp 📷
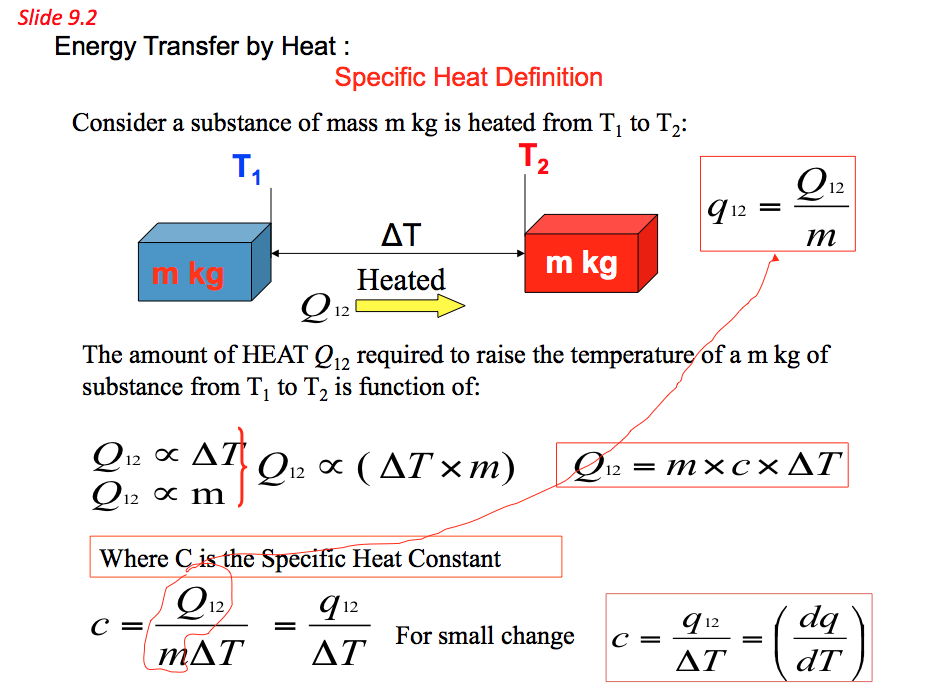

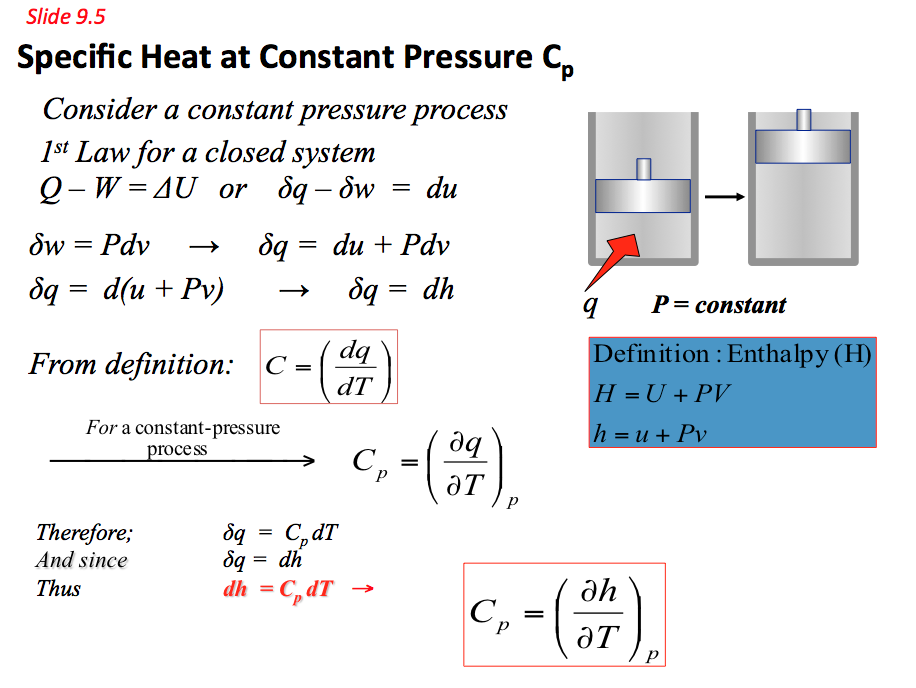
- Specific heat capacity of a substance is defined as the amount of energy required to change the temperature of 1 kg of the substance by 1°C.
-
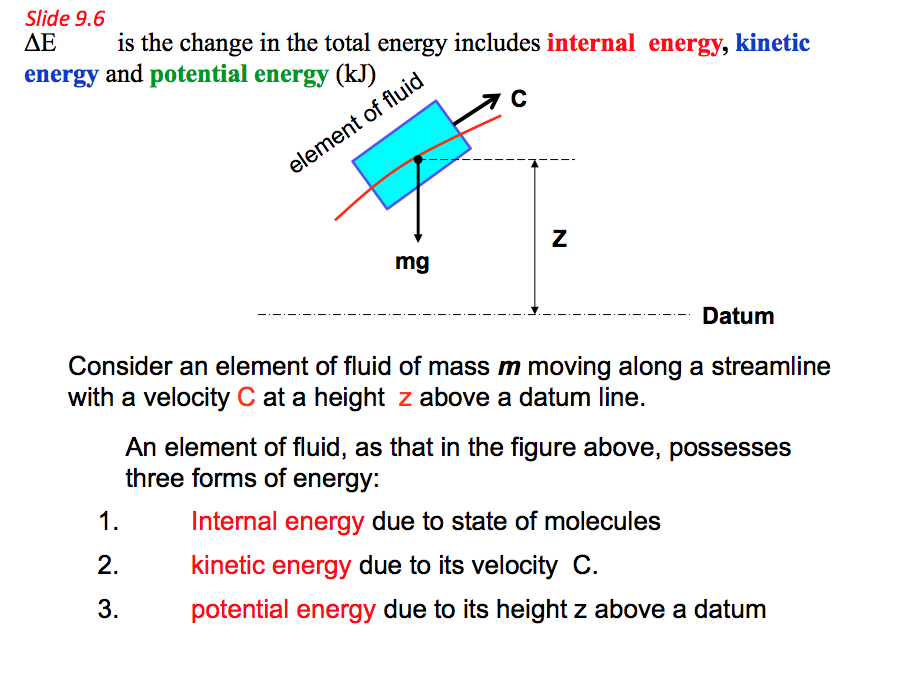
9.6 Internal Energy (U), Kinetic Energy (KE) and Potential Energy (PE).
- For any element of fluid flowing along a streamline above a datum line possesses three forms of energy:
- Energy Change 📷
- 1. Internal energy: When energy is added to a gas it manifests itself at the microscopic level in three forms; namely, vibrational energy, rotational energy and transitional energy. The combination of all these three forms of energy is called Internal Energy of a system and denoted the by the symbol U.
- Internal Energy (U) 📷
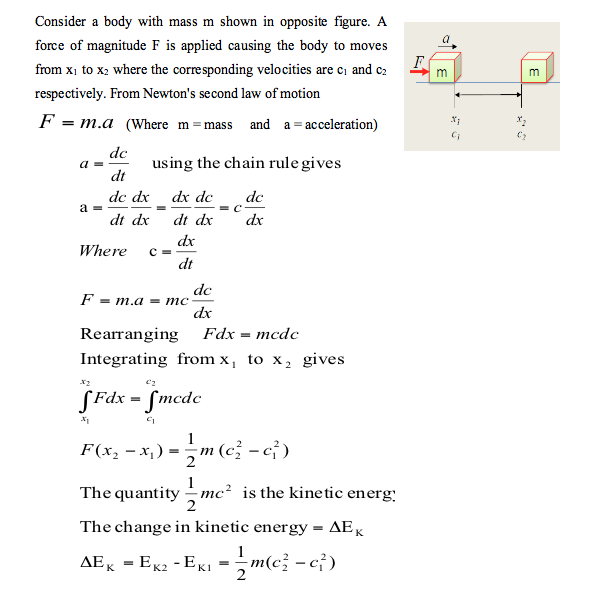
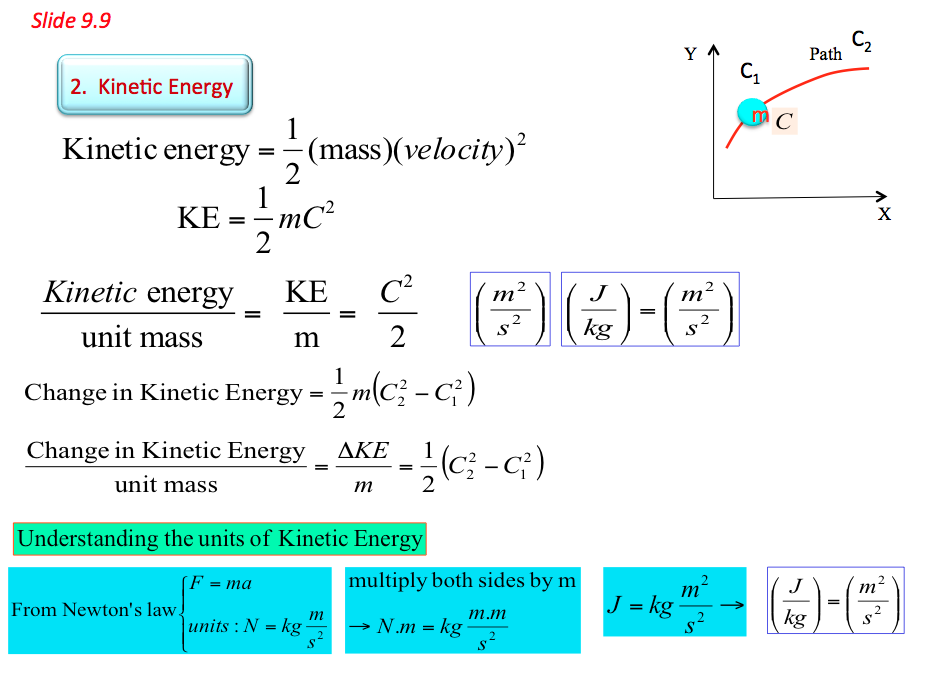
- 2. Kinetic energy and potential energy are macroscopic forms of energy possessed by the system and depend on some outside reference frame. a. Kinetic energy is the name given to the energy an object possesses by virtue of its motion. By combining Newton's laws with our definitions of work, velocity, and acceleration, we can develop a complete definition for kinetic energy:
- Internal Energy Animation 9.8 📹
- Example
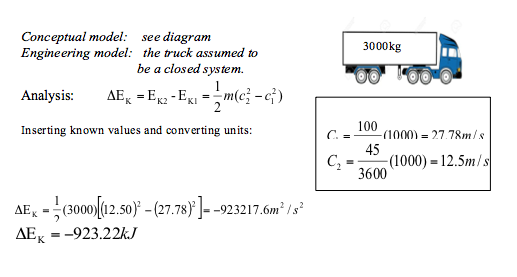
A truck of mass 3000kg initially is traveling at a velocity of 100km/h relative to the road. Given that the vehicle decelerates to a final velocity of 45km/h, determine the change in kinetic energy, in kJ.
Kiinetic Energy 📷
Example
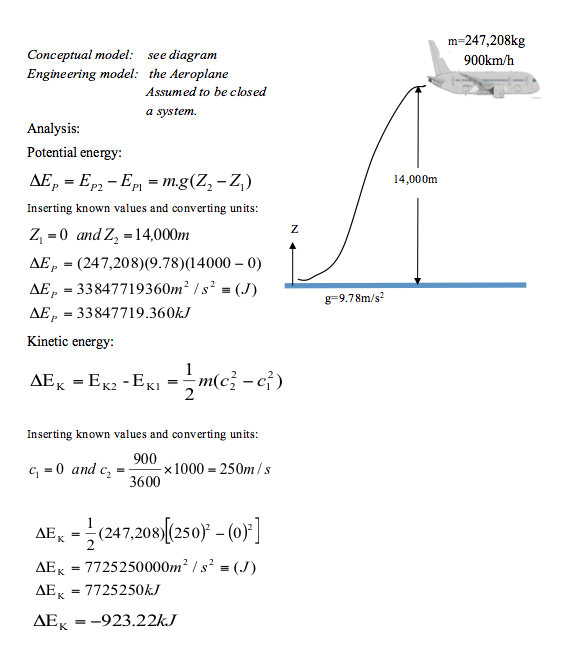
A Boeing 787-9 Dreamliner whose mass is 247,208 kg is flying with a velocity of 900km/h at an altitude of 14,000m, both measured relative to the surface of earth. Given that the acceleration of gravity can be assumed to be constant at 9.78m/s2, determine the potential and kinetic energies of the aeroplane, both in kJ.
- Example
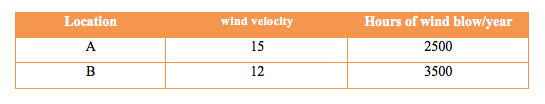
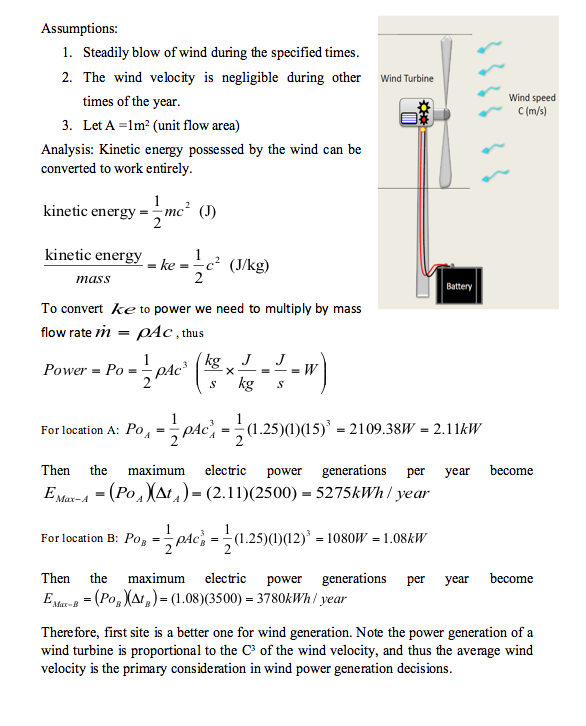
Many factors affecting the selection of a location of wind farm to harness the energy of the wind. These factors can be broadly classified to two categories namely; physical factors and social factors. The most important parameter in the category of physical factors is the wind speed. A company is considering two locations to install a wind farm for wind power generation. Data of wind speed and number of hours of wind blow per year for locations A & B are provided in Table1 below. Neglecting the wind velocity at other times of the year for simplicity, take the density of air ρ = 1.25 kg/m3 and based on the wind speed only determine which a better site is for wind power generation.
Hint: Note that the mass flow rate of air is proportional to wind velocity
-
Thermodynamics: TUTORIAL 9 : ENERGY FORMS
- 1. To determine Cx (the specific heat of material X) a student places 90g of material X in a 50g iron calorimeter that contains 75g of water, all initially at 15oC. Given that when the student added 150g of water at 90oC, the final temperature was measured as 55oC, determine Cx. Take C water = 4.181kJ/kg and C Iron = 0.449kJ/kg.
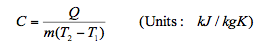
Here we want to make use of conservation of energy, namely the heat absorbed by the original amount of material X, the calorimeter and the initial water is equal to the heat lost by the added hot water. This gives us the equation:
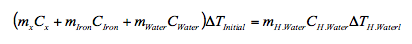
Substitute for values of the specific heats of water and iron and separate the variables gives,
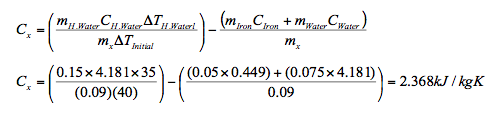
- 2. A racing compact car, mass 642 kg, moving at a speed of 360km/h brakes to a stop. The brake pads are made of 5.5kg carbon-ceramic brake (ccb) that absorbs energy efficiently. Given the specific heat of the brake pads is Cccb = 0.810kJ/kgK, determine the increase in temperature of the brakes.
During braking, the kinetic energy of the car is converted into heat energy. So
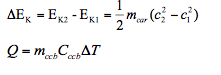
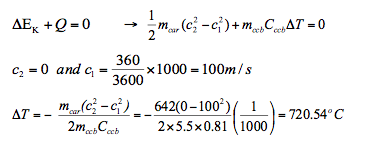
- 1. To determine Cx (the specific heat of material X) a student places 90g of material X in a 50g iron calorimeter that contains 75g of water, all initially at 15oC. Given that when the student added 150g of water at 90oC, the final temperature was measured as 55oC, determine Cx. Take C water = 4.181kJ/kg and C Iron = 0.449kJ/kg.