Alternating Voltages and Currents
- Introduction
- Reactance of Inductors and Capacitors
- Phasor Diagrams
- Impedance
- Complex Notation
Introduction
- From our earlier discussions we know that
where $V_p$ is the peak voltage
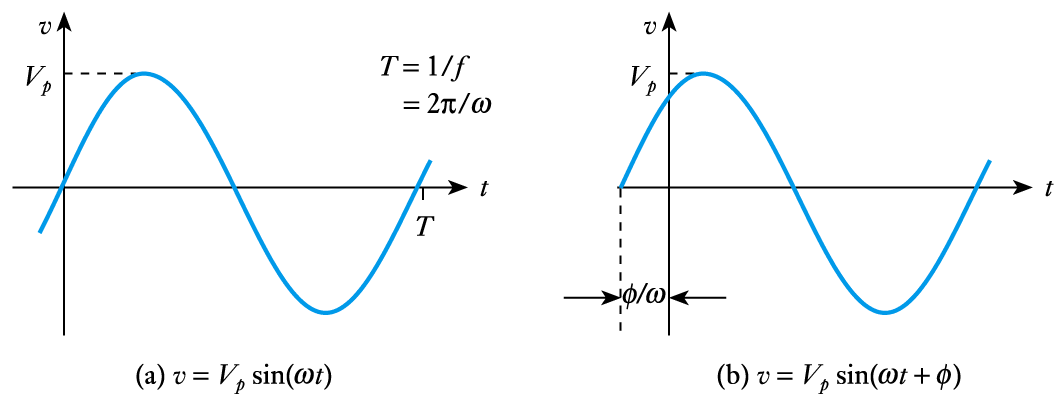
$\large V=V_p \; sin (\omega t + \phi)$
$\omega$ is the angular frequency
$\phi$ is the phase angle- Since $\omega = 2 \pi f$ it follows that the period $T$ is given by
\begin{align*} \large T = \frac{\large 1}{f} =\frac{\large 2 \pi}{\omega} \qquad \qquad \qquad \qquad \qquad \qquad \end{align*}
📷 If $\phi$ is in radians, then a time delay t is given by $\phi / \omega$ (as shown here)
-
- Inductance

$$\frac{\text{Peak value of voltage}}{\text{Peak value of current}} = \frac{\text{Peak value of } (\omega LI _P \text{cos} (\omega t))}{\text{Peak value of } (I _P \text{sin} (\omega t)) } = \frac{\omega LI _P}{I _P} = \omega L$$
- Capacitance
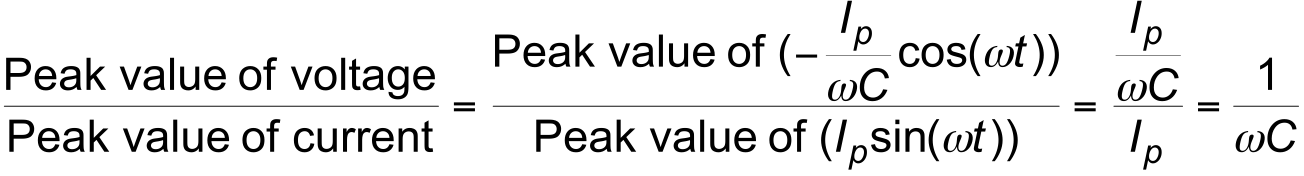
$$\frac{\text{Peak value of voltage}}{\text{Peak value of current}} = \frac{\text{Peak value of } (- \frac{I _P}{\omega C} \text{cos}(\omega t))}{\text{Peak value of } (I _P \text{sin} (\omega t)) } = \frac{\frac{I _P}{\omega C}}{I _P} = \frac{1}{\omega C}$$
- The ratio of voltage to current is a measure of how the component opposes the flow of electricity
- In a resistor this is termed its resistance
- In inductors and capacitors it is termed its reactance
- Reactance is given the symbol $X$
- Therefore
\begin{align*} \text {Reactance of an inductor, }X_L = \omega L \end{align*}
\begin{align*} \text {Reactance of an inductor, }X_C = \frac{1}{\omega C} \end{align*}- Since reactance represents the ratio of voltage to current it has units of ohms
- The reactance of a component can be used in much the same way as resistance:
– for an inductor$V = IX _L$– for a capacitor$V = IX _C$- Example – see Example 6.3 from course text. A sinusoidal voltage of $5 V$ peak and $100\; Hz$ is applied across an inductor of $25 mH$. What will be the peak current?
At this frequency, the reactance of the inductor is given by
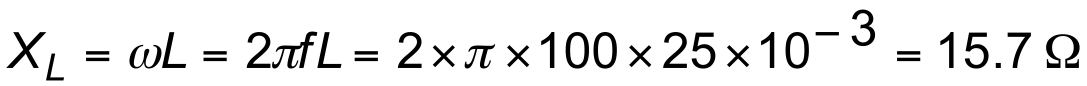
Therefore
$$I_L = \frac{V_L}{X_L} = \frac{5}{15.7} = 318 \; mA \text{ peak}$$
Voltage and Current
- 🎥 Watch a video on alternating voltages and currents - Adobe Flash video
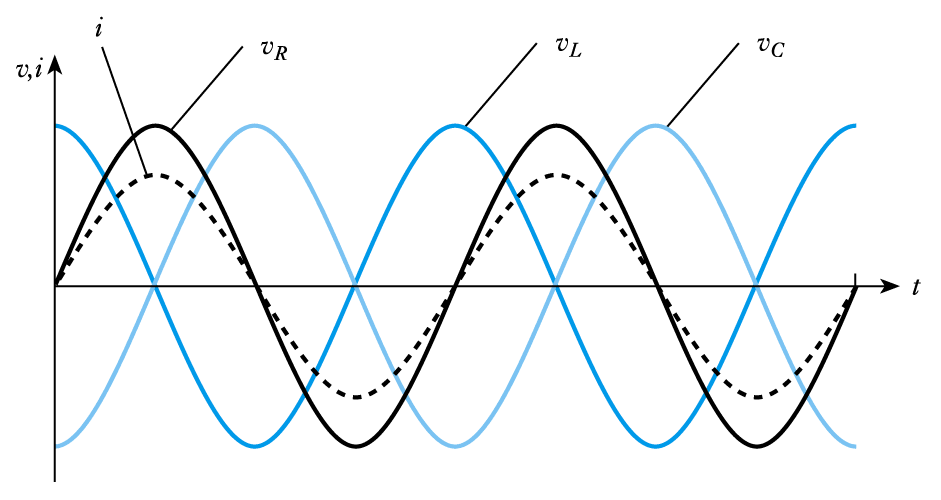
- Consider the voltages across a resistor, an inductor and a capacitor, with a current of
\begin{align*} \large i = I_p\; sin (\omega t) \qquad \qquad \qquad \qquad \qquad \qquad \end{align*}- Resistors
– from Ohm law we know $$V_R = iR$$ – therefore if $i =I_P \; \text{sin} (\omega t)$
$$ V_R = I_PR \; \text{sin} (\omega t) $$
- Inductors - in an inductor
$$V_L = L \frac{di}{dt}$$– therefore if $i=I_P\; sin(\omega t)$
$$ V_L = L\frac{d(I_P \; sin (\omega t))}{dt} = \omega LI _P \; cos (\omega t) $$
- Capacitors - in a capacitor
$$ V_C = \frac{1}{C} \int idt $$– therefore if $i = I _P\; sin (\omega t)$
$$V _C = \frac{1}{C} \int I _P \; sin (\omega t) = - \frac{I _P}{\omega C} \; cos (\omega t)$$
📷 See this diagram
Reactance of Inductors and Capacitors
- Let us ignore, for the moment the phase angle and consider the magnitudes of the voltages and currents
- Let us compare the peak voltage and peak current
- Resistance

$$\frac{\text{Peak value of voltage}}{\text{Peak value of current}} = \frac{\text{Peak value of } (I _P R \text{sin} (\omega t))}{\text{Peak value of } (I _P \text{sin} (\omega t)) } = \frac{I _P R}{I _P} = R$$
- Inductance
-
- 📷 Phasor diagrams can be used to represent the addition of signals. This gives both the magnitude and phase of the resultant signal (can be seen here)
- 📷 Phasor diagrams can also be used to show the subtraction of signals
- 📷 Phasor analysis of an $RL$ circuit
See Example 6.5 in the text for a numerical example
- 📷 Phasor analysis of an $RC$ circuit
See Example 6.6 in the text for a numerical example
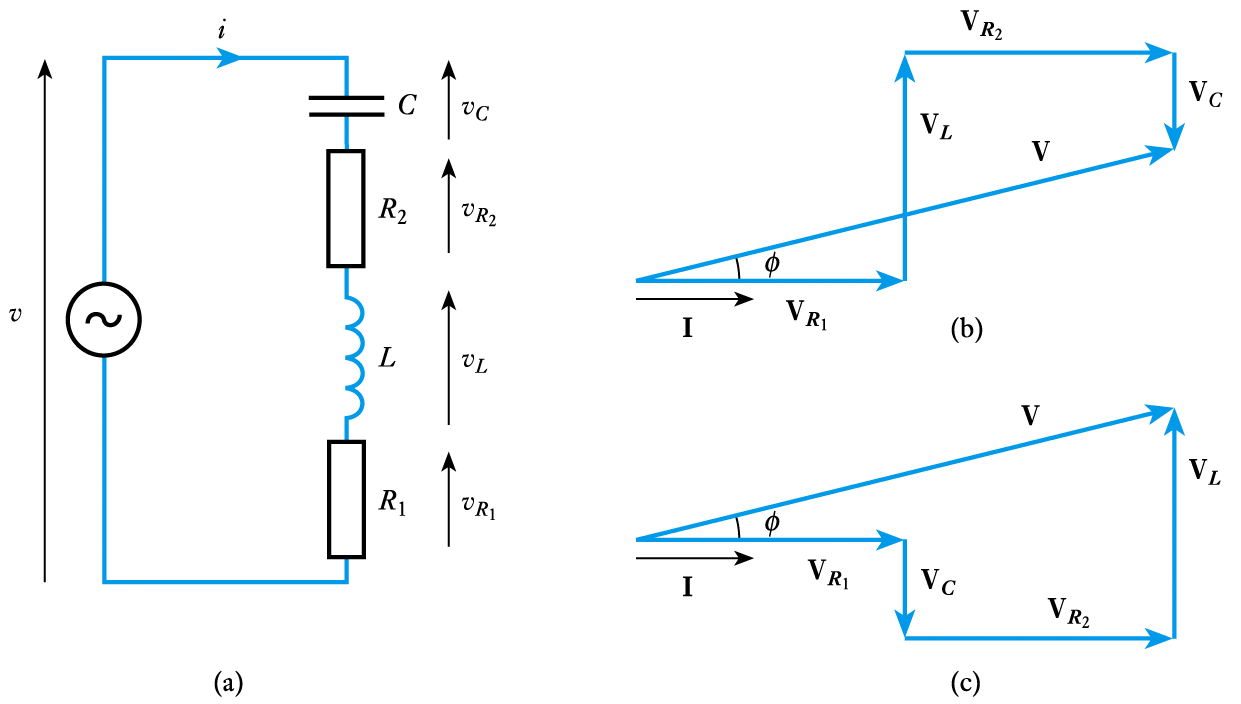
- 📷 Phasor analysis of an $RLC$ circuit
- Phasor analysis of parallel circuits
in such circuits the voltage across each of the components is the same and it is the currents that are of interest
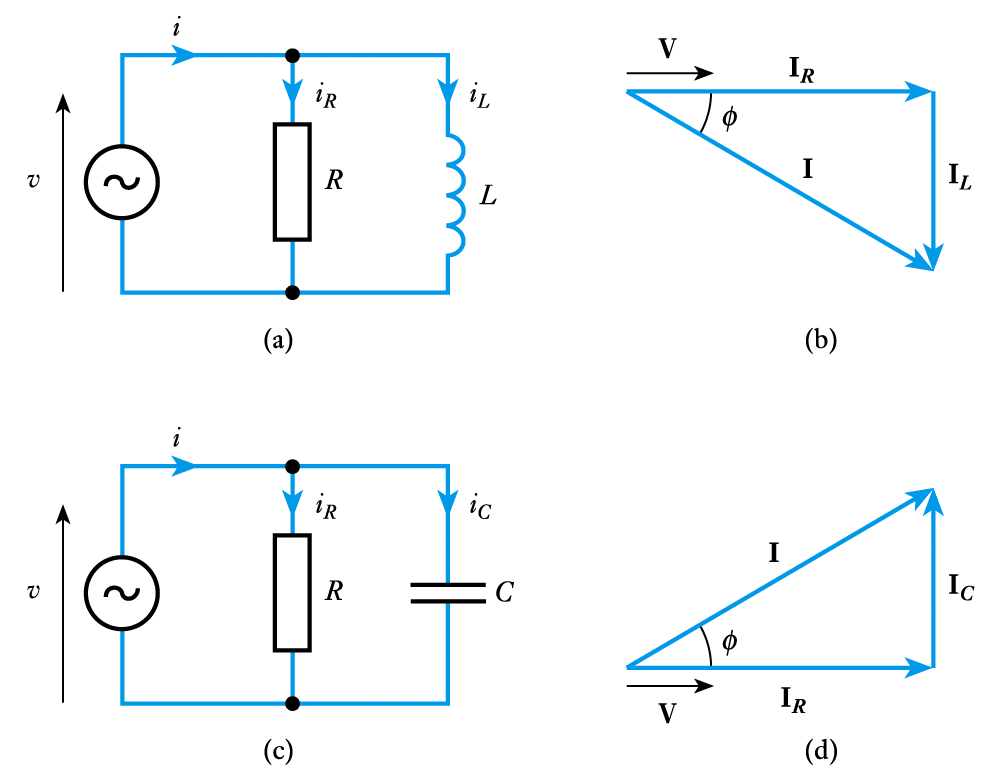
Phasor Diagrams
- Sinusoidal signals are characterised by their magnitude, their frequency and their phase
- In many circuits the frequency is fixed (perhaps at the frequency of the AC supply) and we are interested in only magnitude and phase
- In such cases we often use phasor diagrams which represent magnitude and phase within a single diagram
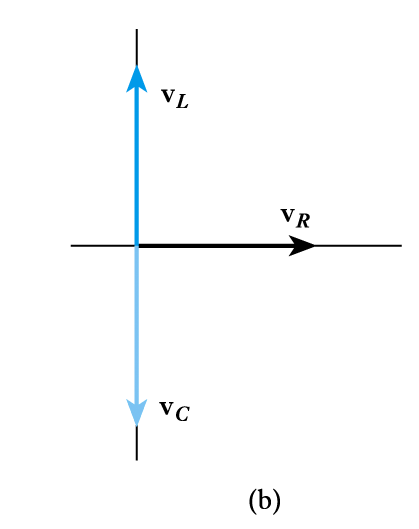
- Examples of phasor diagrams (a) here $L$ represents the magnitude and $ \large \phi$ the phase of a sinusoidal signal
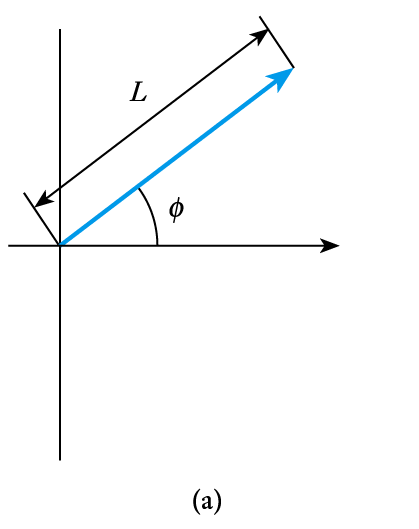
(b) shows the voltages across a resistor, an inductor and a capacitor for the same sinusoidal current
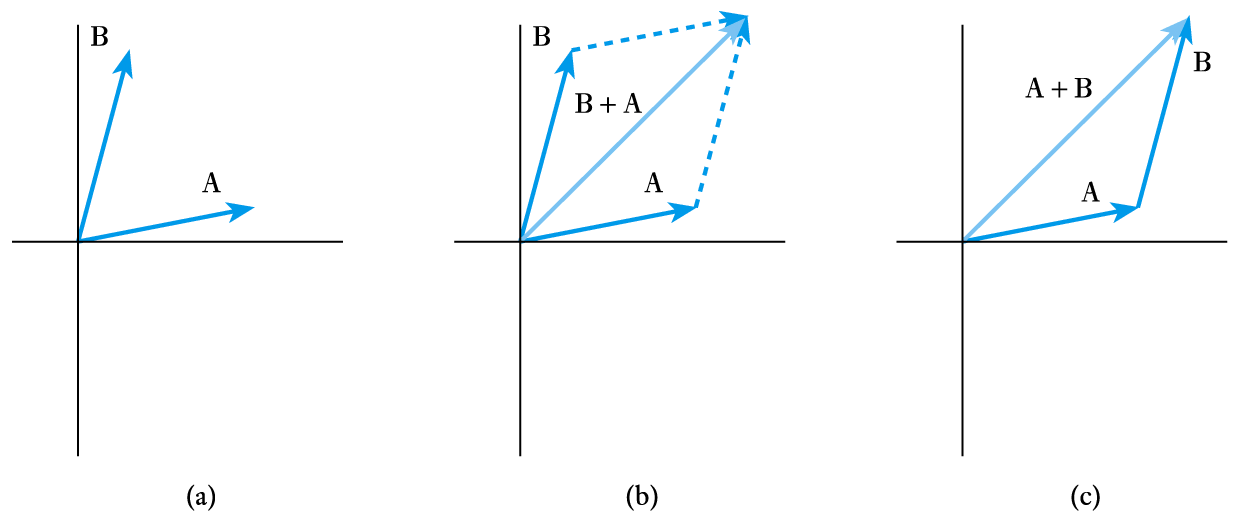
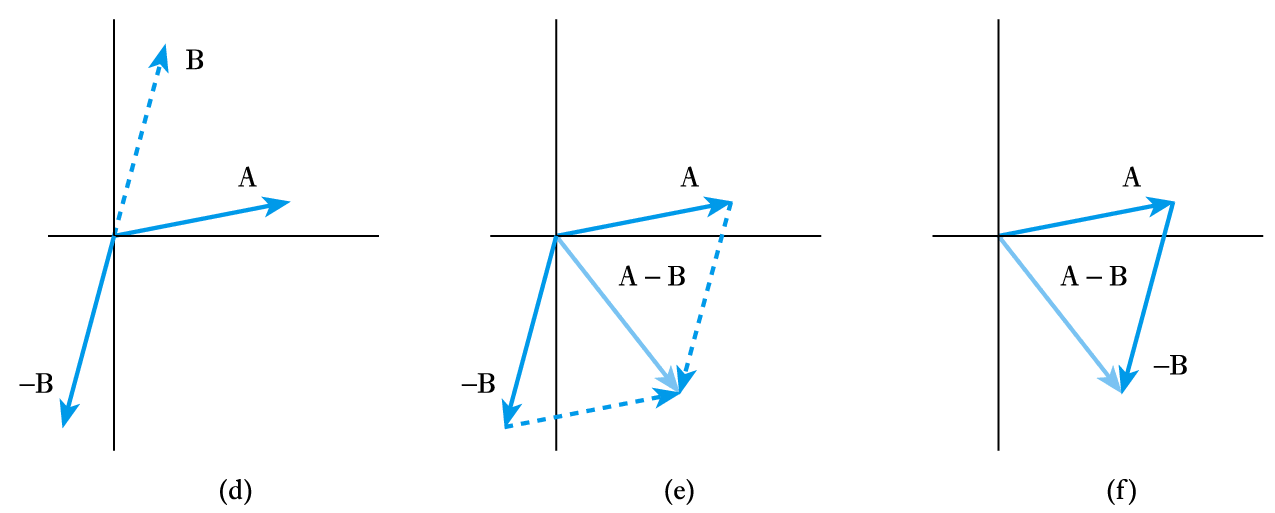
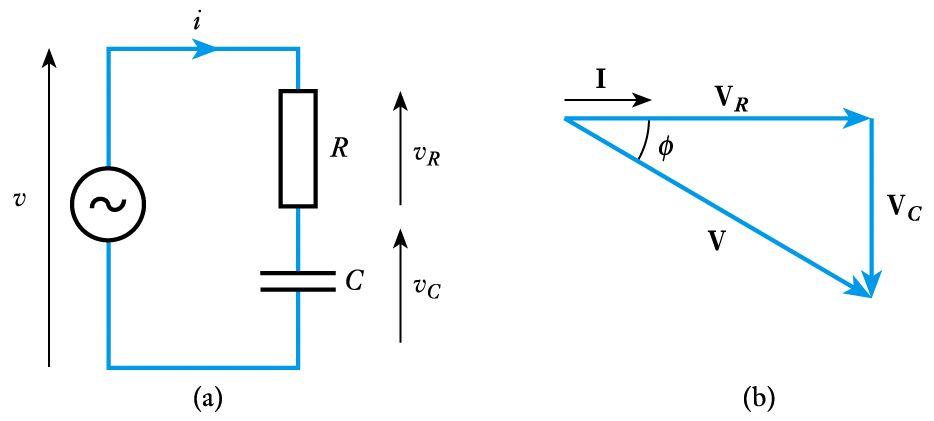
- 📷 Phasor diagrams can be used to represent the addition of signals. This gives both the magnitude and phase of the resultant signal (can be seen here)
-
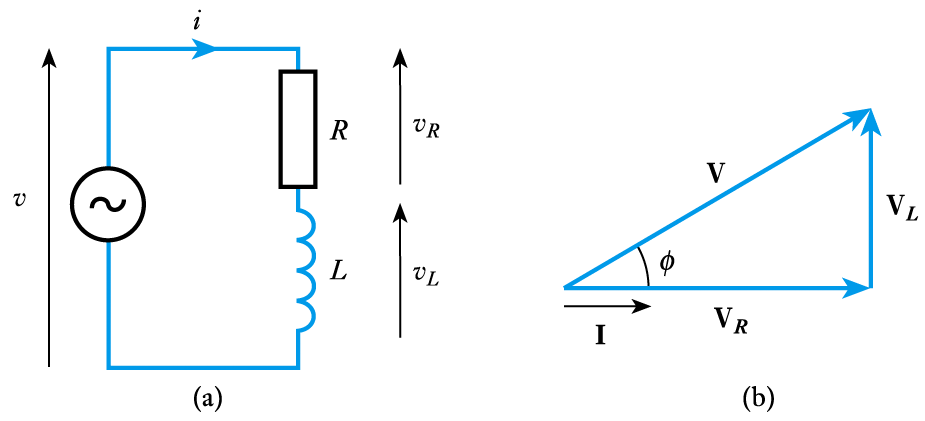
- From the phasor diagram the phase angle of the impedance is given by
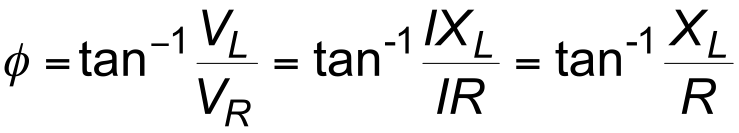 $$\large \phi = \text{tan}^{-1} \frac{V_L}{V_R} = \text{tan} ^{-1} \frac{IX_L}{IR} = \text{tan}^{-1 }\frac{X_L}{R}$$
$$\large \phi = \text{tan}^{-1} \frac{V_L}{V_R} = \text{tan} ^{-1} \frac{IX_L}{IR} = \text{tan}^{-1 }\frac{X_L}{R}$$
- This circuit contains an inductor but a similar analysis can be done for circuits containing capacitors
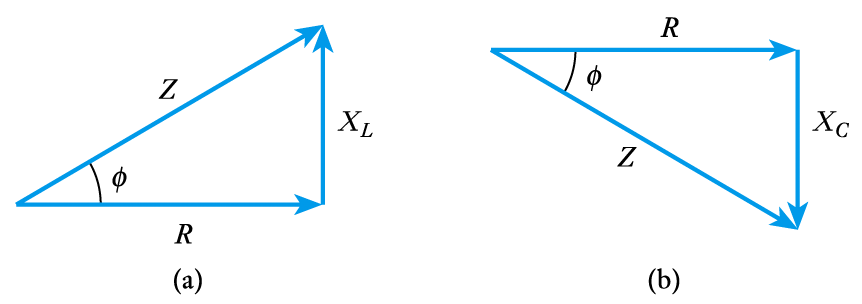
- In general
\begin{align*} Z = \sqrt{R^2 = X^2} \end{align*}$$\text{And}$$\begin{align*} \phi = \text{tan} ^{-1} \frac{X}{R} \end{align*}
- A graphical representation of impedance
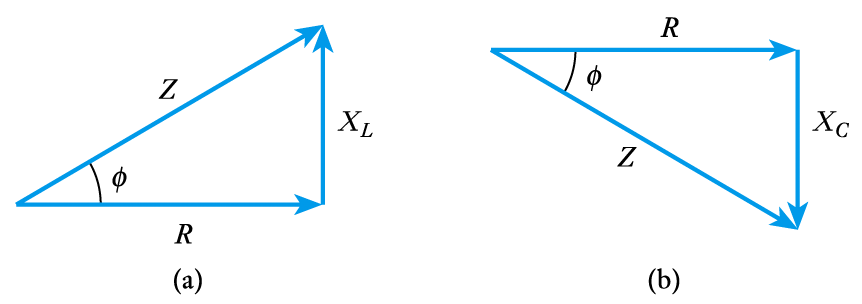
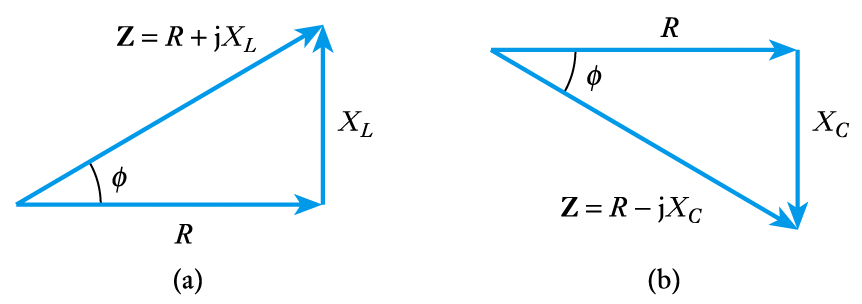
Impedance
- In circuits containing only resistive elements the current is related to the applied voltage by the resistance of the arrangement
- In circuits containing reactive, as well as resistive elements, the current is related to the applied voltage by the impedance, $Z$ of the arrangement
– this reflects not only the magnitude of the current but also its phase
– impedance can be used in reactive circuits in a similar manner to the way resistance is used in resistive circuits
- Consider the following circuit and its phasor diagram
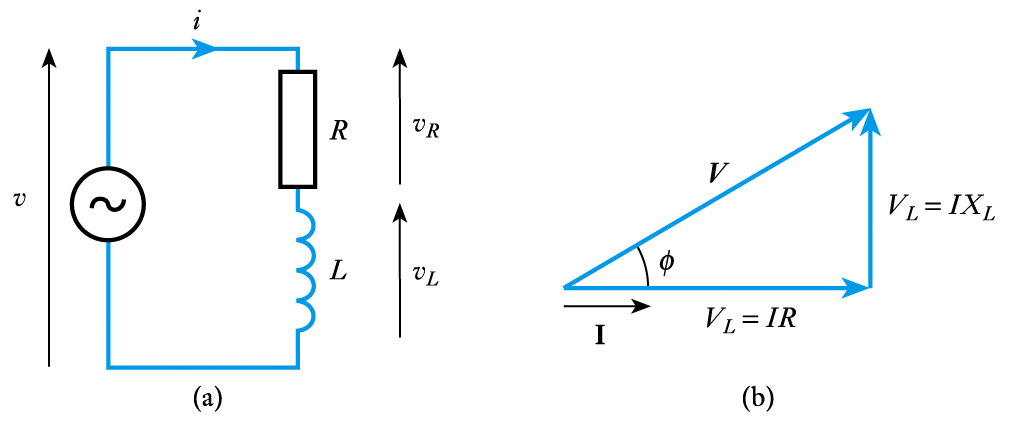
- From the phasor diagram it is clear that that the magnitude of the voltage across the arrangement $V$ is
\begin{align*} V =& \sqrt{V_R \; ^2 + V_L \; ^2} \\ =& \sqrt{(I R)^2 + (I X_L)^2} \\ =& I \sqrt{R^2 + X_L \; ^2} \\ =& I Z \\ \text{ Where} \\ Z =& \sqrt{R^2 + X_L \;^2} \end{align*}
- $Z$ is the magnitude of the impedance, so $Z =$|$Z$|

- From the phasor diagram the phase angle of the impedance is given by
-
- Manipulating complex impedances
– complex impedances can be added, subtracted, multiplied and divided in the same way as other complex quantities
– they can also be expressed in a range of forms such as the rectangular, polar and exponential forms– if you are unfamiliar with the manipulation of complex quantities (or would like a little revision on this topic) see Appendix D of the course text which gives a tutorial on this subject
- Example – see Example 6.7 in the course text.
Determine the complex impedance of this circuit at a frequency of $50\; Hz$
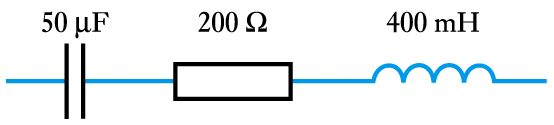
- At 50Hz, the angular frequency $\omega = 2 \pi f = 2 \times \pi \times 50 =314 \text{ rad/s}$
Therefore
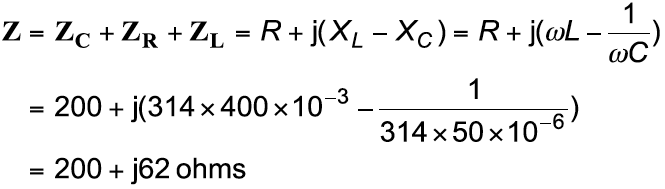
- Using complex impedance
(Example continued on next screen).
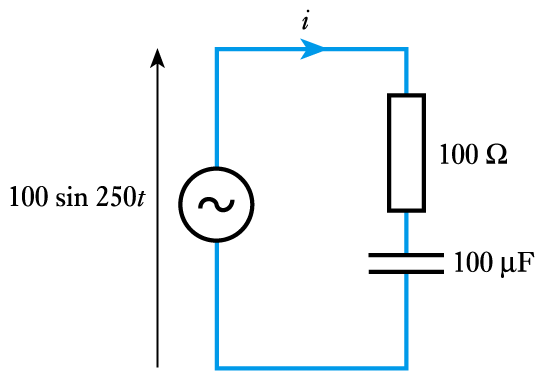
Complex Notation
- Phasor diagrams are similar to Argand Diagrams used in complex mathematics
- We can also represent impedance using complex notation where
- Resistors: $Z_R = R$
- Inductors: $Z_L = jX_L = j \omega L$
- Capacitors: $ Z_c = -jX_C = -j\frac{1}{\omega C} = \frac{1}{j \omega C}$
- Graphical representation of complex impedance

- Series and parallel combinations of impedances
– impedances combine in the same way as resistors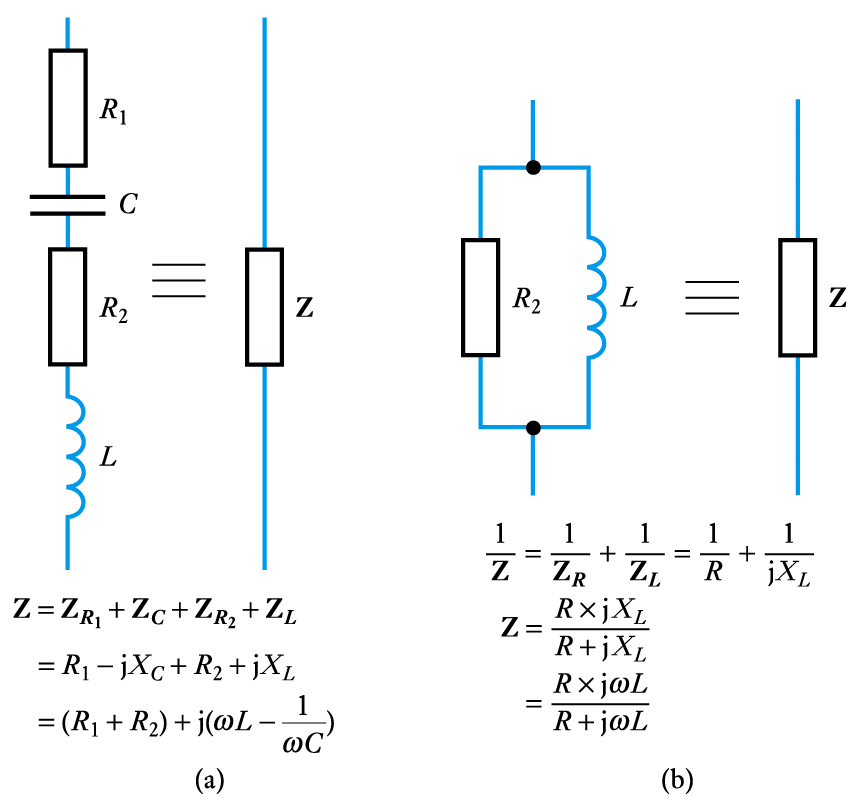


- Manipulating complex impedances
-
- A further example
A more complex task is to find the output voltage of this circuit The analysis of this circuit, and a numerical example based on it, are given in Section 6.6.4 and Example 6.8 of the course text.
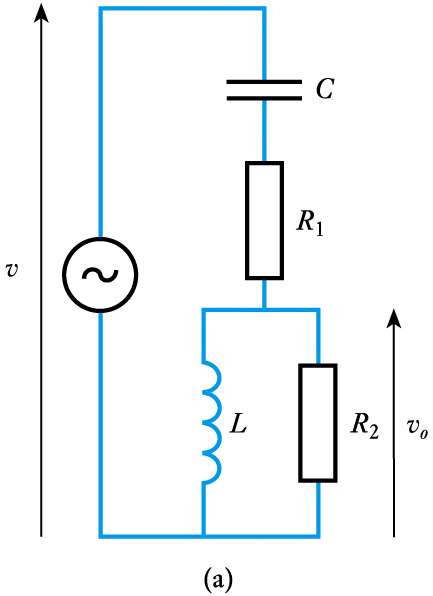
(Cont.)
- Example – see Section 6.6.4 in course text Determine the current in this circuit Since $v = 100 \text{ sin } 250 t$ , then $\omega = 250$
Therefore
\begin{align*} Z =& R - jX_C \\ =& R - j \frac{1}{\omega C} \\ =& 100 - j \frac{1}{250 \times 10 ^{-4}} \\ =& 100 - j 40 \end{align*}
- The current is given by $v$/$Z$ and this is easier to compute in polar form
\begin{align*} Z =& 100 - j40 \\ |Z| =& \sqrt{100^2 + 40^2} - 107.7 \\ \angle{Z}=& \text{ tan} ^{\large -1} \frac{ - 40}{100} = -21.8 ^\circ \\ Z=& 107.7 \angle{} - 21.8^\circ \end{align*}
- Therefore
\begin{align*} i = \frac{v}{Z} = \frac{100 \angle{0} }{107.7 \angle{} - 21.8} = 0.93 \angle{21.8^\circ} \end{align*} - A further example
-
Further Study
- 🎥 Watch a video on using complex impedance - Adobe Flash video
- The Further Study section at the end of Chapter 6 looks at the characteristics of a simple reactive circuit.
- See if you can model the behaviour of the circuit at a single frequency and then watch the video to check your analysis.
Key Points
- A sinusoidal voltage waveform can be described by the equation $ v = V _P \text{ sin } (\omega t + \phi)$
- The voltage across a resistor is in phase with the current, the voltage across an inductor leads the current by 90°, and the voltage across a capacitor lags the current by 90°
- The reactance of an inductor $X_L = \omega L$
- The reactance of a capacitor $X_C = 1/ \omega C$
- The relationship between current and voltage in circuits containing reactance can be described by its impedance
- The use of impedance is simplified by the use of complex notation
-
- 6.10 If a sinusoidal current is passed through a resistor, what is the phase relationship between this current and the voltage across the component?
- 6.11 If a sinusoidal current is passed through a capacitor, what is the phase relationship between this current and the voltage across the component?
- 6.12 If a sinusoidal current is passed through an inductor, what is the phase relationship between this current and the voltage across the component?
- 6.13 How can the word ‘civil’ assist in remembering the phase relationship between currents and voltages in inductors and capacitors?
- 6.14 Explain what is meant by the term ‘reactance’.
- 6.15 What is the reactance of a resistor?
- 6.16 What is the reactance of an inductor?
- 6.17 What is the reactance of a capacitor?
- 6.18 Calculate the reactance of an inductor of 20 $mH$ at a frequency of $100 \; Hz$, being sure to include the units in your answer.
- 6.19 Calculate the reactance of a capacitor of 10 $nF$ at an angular frequency of 500 rad /s, being sure to include the units in your answer.
- 6.20 A sinusoidal voltage of 15 $V$ r.m.s. at $250\; Hz$ is applied across a 50 $\mu F$ capacitor. What will be the current in the capacitor?
- 6.21 A sinusoidal current of 2 $mA$ peak at 100 rad/s flows through an inductor of 25 $mH$. What voltage will appear across the inductor?
- 6.22 Explain briefly the use of a phasor diagram.
- 6.23 What is the significance of the length and direction of a phasor?
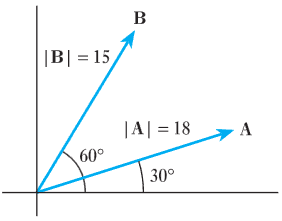
- 6.24 Estimate the magnitude and phase of ( $A + B$ ) and ( $A − B$ ) in the following phasor diagram.
- ⬇Download chapter 6 tutorial
Exercises
- 6.1 A signal v is described by the expression $v = 15 \text{ sin } 100t$. What is the angular frequency of this signal, and what is its peak magnitude?
- 6.2 A signal v is described by the expression $v = 25 \text{ sin } 250 t$ . What is the frequency of this signal (in Hz), and what is its r.m.s. magnitude?
- 6.3 Give an expression for a sinusoidal signal with a peak voltage of $20 \; V$ and an angular frequency of $300$ rad/s.
- 6.4 Give an expression for a sinusoidal signal with an r.m.s. voltage of $14.14 V$ and a frequency of $50\; Hz$.
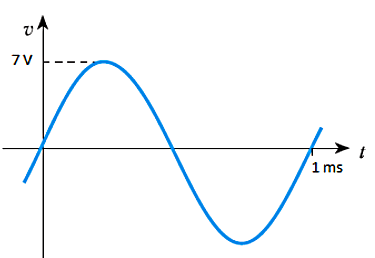
- 6.5 Give an expression for the waveform shown in the following diagram.
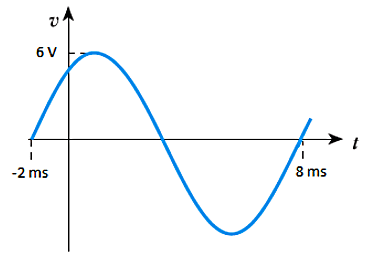
- 6.6 Give an expression for the waveform shown in the following diagram.
- 6.7 Give an expression relating the voltage across a resistor to the current through it.
- 6.8 Give an expression relating the voltage across an inductor to the current through it.
- 6.9 Give an expression relating the voltage across a capacitor to the current through it.
-
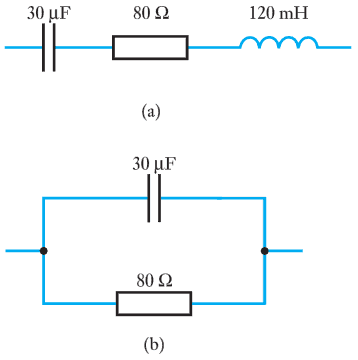
- 6.34 Determine the complex impedance of the following arrangements at a frequency of $100\; Hz$.
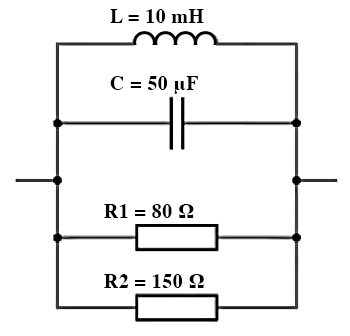
- 6.35 Use your answer to Exercise 6.34 to devise a simpler arrangement of components that would have a similar behaviour to the circuit of Exercise 6.34 at a frequency of $100\; Hz$.
- 6.36 Repeat the calculations of Exercise 6.34 assuming that the circuit will be used at a frequency of $200\; Hz$.
- 6.37 Use your answer to Exercise 6.36 to devise a simple arrangement of components that would have a similar behaviour to the circuit of Exercise 6.34 at a frequency of $200\; Hz$.
- 6.38 Express $x = 20 + j30$ in polar form and in exponential form.
- 6.39 Express $y = 25 \angle{} − 40^\circ$ in rectangular form and in exponential form.
- 6.40 A voltage $v = 60 \text{ sin } 314 t$ is applied across a series combination of a $10 \Omega$ resistor and an inductance of $50 mH$. Determine the magnitude and phase of the resulting current.
- 6.41 A current of $i = 0.5 \text{ sin } 377 t$ is passed through a parallel combination of a resistance of $1 k \Omega$ and a capacitance of $5 \mu F$. Determine the magnitude and phase of the resulting voltage across the combination.
- ⬇ Tutorial Solutions
Exercises (cont.)
- 6.25 A voltage is formed by summing two sinusoidal waveforms of the same frequency. The first has a magnitude of $20 V$ and is taken as the reference phase (that is, its phase angle is taken as 0°). The second has a magnitude of $10 V$ and leads the first waveform by 45°. Draw a phasor diagram of this arrangement and hence estimate the magnitude and phase of the resultant signal.
- 6.26 A sinusoidal current of 3 A at $100\; Hz$ flows through a series combination of a resistor of 25 $\Omega$ and an inductor of $75 mH$. Use a phasor diagram to determine the voltage across the combination and the phase angle between this voltage and the current.
- 6.27 A sinusoidal voltage of $12 V$ at $500\; Hz$ is applied across a series combination of a resistor of $5 k \; \Omega$ and a capacitor of $100 nF$. Use a phasor diagram to deter- mine the current through the combination and the phase angle between this current and the applied voltage.
- 6.28 Use a phasor diagram to determine the magnitude and phase angle of the impedance formed by the series combination of a resistance of 25 $\Omega$ and a capacitance of $10 \mu F$, at a frequency of $300\; Hz$.
- 6.29 If $x = 5 + j7$ and $y = 8 − j10$, evaluate $( x + y ), ( x − y ), ( x \times y )$ and $( x \div y )$.
- 6.30 What is the complex impedance of a resistor of $1 k \Omega$ at a frequency of $1 \; kHz$?
- 6.31 What is the complex impedance of a capacitor of $1 \mu F$ at a frequency of $1 \; kHz$?
- 6.32 What is the complex impedance of an inductor of $1 mH$ at a frequency of $1 \; kHz$?
- 6.33 Determine the complex impedance of the following arrangements at a frequency of $200\; Hz$.